Taxol

ABSTRACT Mobility of taxol inside microtubules was investigated using fluorescence recovery after photobleaching on flow-aligned bundles. Bundles were made of microtubules with either GMPCPP or GTP at the exchangeable site on the tubulin dimer. Recovery times were sensitive to bundle thickness and packing, indicating that taxol molecules are able to move laterally through the bundle. The density of open binding sites along a microtubule was varied by controlling the concentration of taxol in solution for GMPCPP samples. With >63% sites occupied, recovery times were independent of taxol concentration and, therefore, inversely proportional to the microscopic dissociation rate, k^sub off^. It was found that 10 k^sup GMPCPP^^sub off^ [asymptotically =] k^sup GTP^^sub off^, consistent with, but not fully accounting for, the difference in equilibrium constants for taxol on GMPCPP and GTP microtubules. With

INTRODUCTION

It has long been known (Berg, 1978) that reversible binding can alter the apparent kinetics of a chemical reaction (for a review see Sung et al, 1997). Much of the interest in this subject comes from the ability of reversible binding to reduce the dimensionality of a diffusive search, and to thereby enhance the rate of a biochemical reaction (Adam and Delbruck, 1968). This phenomenon, known as facilitated diffusion, is mostly studied on planar substrates (Edelstein and Agmon, 1997; Lagerholm and Thompson, 1998), for applications to cell signaling (Thompson and Axelrod, 1983) and biosensors (Ramakrishnan and Sadana, 1999). On linear substrates, the classic example is Escherichia coli lac repressor rapidly binding to its specific sequence on [lambda]-DNA (Riggs et al, 1970; Berg et al., 1981; Vonhippel and Berg, 1989; Winter and Vonhippel, 1981). Facilitated diffusion has since been demonstrated and quantified for a variety of DNA binding proteins (Dowd and Lloyd, 1990; Herendeen et al, 1992; Jack et al., 1982; Nardone et al, 1986; Ricchetti et al., 1988; Surby and Reich, 1996).

Reversible binding may also lead to facilitated diffusion along the network of microtubules inside eukaryotic cells. Microtubules are hollow, cylindrical lattices of tubulin protein (Alberts et al., 1994). Each tubulin dimer has binding sites for a variety of biologically important ligands, including motor proteins (Heald and Nogales, 2002; Mandelkow and Johnson, 1998; Sosa et al., 1997; Drewes et al., 1998), microtubule-associated proteins (Heald and Nogales, 2002; Drewes et al., 1998), and therapeutic drugs (Downing, 2000). The linear geometry, extraordinary stiffness, and close proximity of numerous binding sites make microtubules both interesting and particularly accessible for one-dimensional transport experiments. In this article, we assess the influence of reversible binding on the diffusion of the therapeutic drug taxol.

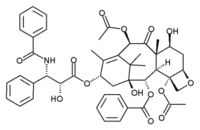
Taxol is the generic name for Paclitaxel, a microtubule-stabilizing drug important in cancer therapy (Parekh and Simpkins, 1997). Taxol binds to a site located on the inner surface of the microtubule wall (Nogales et al., 1999), where it is thought to enhance lateral contacts between dimers (Downing, 2000). Since it is small (1 nm) compared to the inner diameter of a microtubule ( 16 nm), it is expected that reversible binding, more than steric hindrance, should hinder diffusion of taxol along the microtubule axis. The effect should be especially pronounced at low taxol concentrations if the microtubule wall is impermeable (Odde, 1998). Observations of rapid association and equilibration of taxol on microtubules (Evangelio et al., 1998) originally cast doubt on the assignment of the taxol binding site to the microtubule interior. However, recent studies have shown that microtubule walls have 1- to 2-nm fenestrations that could allow small molecules, like taxol, to pass (Nogales et al, 1999; Meurer-Grob et al., 2001). How these pores affect mobility along the microtubule axis is an open question with implications for the biological relevance of the microtubule interior.

Here, we investigate taxol mobility using fluorescence recovery after photobleaching (FRAP) of a fluorescently labeled taxol derivative on two different kinds of microtubules: those that, like microtubules in vivo, have hydrolyzed the molecule of GTP at the E-site of each tubulin dimer (GTP microtubules), and those that have a non-hydrolyzable analog of GTP at the E-site (GMPCPP microtubules). These two types of microtubules are known to have equilibrium dissociation constants (Li et al., 2000) for taxol and its various derivatives that differ by two orders of magnitude, 220 K^sup GMPCPP^^sub D^ [asymptotically =] K^sup GTP^^sub D^. Our results confirm that the microtubule wall is permeable to taxol, and show that the fluorescence recovery times decrease with increasing taxol concentration on both types of microtubules.

fluorescence intensity was measured in the center of large beads and compared to a set of standards with botax at known concentration (see FRAP Apparatus and Experimental Procedures for details).

Flow cell preparation and bundle alignment

The flow cell was made of a 25 mm x 75 mm slide, a 24 mm x 60 mm coverslip, a parafilm gasket, and G-75 Sephadex beads (particle size 40-120 [mu]M; Sigma). As Fig. 1 shows, a pair of 2-mm holes were drilled into the glass slide 45 mm apart. The slide and coverslip were cleaned and coated with dextran by being immersed in a 4-mg/ml solution of 110 kD dextran and blown dry. A 5 mm x 50 mm rectangle was cut from the center of a 24 mm x 60 mm piece of parafilm wax and placed on the slide so as to define a flow path between the two holes. Sephadex beads were sprinkled in the flow path to make obstacles that would catch microtubules while flowing. The coverslip was placed on top, and the flow cell was sealed by heating on a hot plate at 80[degrees]C to melt the parafilm wax.

While heating, pressure was applied to the cell to flatten the flow cell and melt the Sephadex beads. The cell was heated slide-side-down to preferentially melt the beads to the slide surface. This ensured that the flowing bundles would wrap around the beads at a point close to the coverslip, within the working distance of the objective.

The cell was loaded by pipetting 10 [mu]l of sample into one of the holes. Another 10 [mu]l of unlabeled taxol at the desired final concentration in GTP(GMPCPP)-PEM-dex was added to the cell to improve alignment and reduce background. The 2-mm holes were sealed with wax so that the sample could be observed for several days. GMPCPP microtubule bundles were 200-800 [mu]M long and were stable for up to a week. GTP microtubule bundles were 200-1200 [mu]m long, and their stability ranged from 4 h to 2 days, depending on the taxol concentration used.

FRAP apparatus and experimental procedures

Experiments were performed on an inverted microscope (Olympus XI70, Tokyo, Japan) outfitted for differential interference contrast microscopy (DIC) and epi-fluorescence. As Fig. 2 shows, the scope was equipped with Hg arc lamps on both transmitted and epi-illumination paths. Transmitted light was focused onto the sample by a water-coupled 60x objective (NA 0.9) used as a condenser. The field iris (aperture) was used to define the bleach spot. DIC was used to locate bundles and position the bleach spot. For DIC, a diffuser, a neutral density filter (ND 0.25), and a blue interference filter (488 nm) were placed in the light path so as not to bleach the sample. For bleaching, a green filter (550 nm) replaced lhe diffuser and the blue filter. A shutter was used to accurately time the bleach exposure, and a second shutter was used to protect the intensified charge-coupled device during bleaching to avoid damaging the phosphorous screen.

Fluorescence recovery after photobleaching was observed by epi-illumination. A shutter and two neutral density filters (ND 0.1 and 0.5) were used to limit bleaching during observation. Light passed through a green excitation filter (535 nm, width 45 nm) for rhodamine and reflected off of a dichroic mirror (560 DRLP). A 60x oil -coupled objective (NA 1.4) focused the light onto the sample, exciting the BODIPY dye (peak 564 nm). The same objective collected the BODIPY emission light (peak 570 nm), and passed it through the dichroic mirror and a red emission filter (630 nm, width 30 nm) to an intensified charge-coupled device camera (DVC 1312 Intensicam, DVC, Austin, TX).

After an appropriate bundle was located by DIC, the bundle was viewed in epi-fluorescence to establish a proper exposure time. DIC was used again to focus and align the bleach spot by closing the aperture and focusing the condenser. Pictures were taken at each step to document the qualities of the bundle and spot. The shutter in the epi-illumination path was adjusted for the correct exposure time and synchronized with the camera's recording intervals. Images were saved directly to RAM by the computer (C-view, DVC). Typical runs required 350 frames (~1.2 Gb) and lasted 30 min to 2 h. Multiple runs were taken on different bundles in the same sample.

In situ measurement of taxol concentration in GTP microtubule samples

After centrifugation and resuspension, GTP microtubules will depolymerize, causing the taxol concentration in solution to increase. To find the actual taxol concentration in each sample, an in situ measurement based on the fluorescence intensity was made.

A set of standard samples with known botax concentrations were prepared for calibration purposes. Each standard is a flow cell containing Sephadex beads and no microtubules, filled with a known concentration of botax ranging from 500 pM to 5 [mu]M. In each standard, Sephadex beads were viewed in epi-fluorescence. Pictures were taken at varying exposure times with the gain settings, magnification, and filters left unchanged. The average intensity of the bead was plotted as a function of exposure time. A linear fit was used to extract the exposure time, t^sub 1/2^, at which the average intensity of the field of view equaled half the maximum intensity, I^sub max^/2, of the camera's dynamic range. The procedure was repeated for several beads in the same standard, and the resultant t^sub 1/2^ values were averaged. Taxol concentration was plotted as a function of average t^sub 1/2^ and found to obey: [TAX] [is proportional to] [left angle bracket]t^sub 1/2^[right angle bracket]^sup -2.0+ or -0.4^

Samples of GTP microtubules were prepared with 100% botax and viewed in epi-fluorescence with the same gain settings, magnification, and filters as with standards. Data was taken and analyzed on large beads in the same manner as with the standards. The taxol concentration of each sample was found using the power law for taxol concentration (above).

Photodamage

Photobleaching of botax could result in photodamage of microtubules if the light intensity is too high or the exposure time is too long, as with other fluorescent compounds (Vigers et al., 1988). Photodamage was easily identified in DlC and fluorescence. In the damaged region, microtubules were destroyed, and fluorescence recovery did not occur. Only data runs where the bundle did not change appearance over the time of the experiment were used. If a bundle moved, shifted, disassembled, or showed signs of photodamage, the data was not used (see Results).

Data analysis

Images were analyzed using NIH Image (http://rsb.info.nih.gov/nih-image/). For each bundle, a rectangular region of interest (ROI) was selected and intensities were averaged over the short dimension and plotted over the long dimension to give an intensity profile (Fig. 3 A). The first image of a time series served as a baseline that was subtracted from each intensity profile to correct for permanent intensity variations along the ROI (not due to the bleach spot). The corrected profiles were fit to a Gaussian curve using the equation:

where a is the vertical offset, b is the amplitude, c is the horizontal offset, and d is the standard deviation (Fig. 3 B). All four parameters were recorded for each time step. The procedure was automated in KaleidaGraph, v. 3.51 (Synergy, Reading, PA) using Apple Script (Apple Computers, Cupertino, CA).

Recovery of fluorescence was measured by fitting the decrease in amplitude over time to an exponential decay of the form:

where [tau] is the characteristic time for decay (Fig. 3 C). Data were filtered using a script that systematically masked amplitudes based on the percent error. Each masked data set was plotted and fit to an exponential decay. The recovery time that minimized the error in [tau] was used.

RESULTS

Video-FRAP experiments

We observed the effect of unoccupied binding sites on taxol mobility in microtubules using fluorescence recovery after photobleaching (FRAP). The experimental design and data analysis used in this study were specially developed for microtubule bundles in a thick sample. Conventional FRAP experiments use a laser to bleach and observe a thin sample of isotropic media (Axelrod et al., 1976). We used video-FRAP (Kapitza et al., 1985) that takes advantage of spatial imaging to correct for bleaching of the fluorophore during observation (see Data Analysis). Previous studies have used the two-dimensional Fourier transform of video-FRAP images to find the diffusion constant in thick samples of isotropic media (Berk et al., 1993; Tsay and Jacobson, 1991). This technique could not be applied to microtubule samples, which were both inhomogeneous and anisotropic. Instead, we used imaging to examine the changing intensity profile along the bundle and extract a characteristic fluorescence recovery time, [tau], from the changing amplitude (Fig. 3). It was not possible to accurately derive diffusion constants directly from the measured recovery times, due to the inhomogeneous distribution of microtubules in three dimensions. Nevertheless, the [tau] values should be inversely proportional to the diffusion constant (Kao et al., 1993).

We confirmed the robustness of our method by repeatedly bleaching a bundle (Fig. 4 A). Recovery times routinely agreed for multiple bleaches at the same location, indicating that bleaching did not damage the microtubule or the taxol binding site. In cases where photodamage did occur, fluorescence never recovered (see Materials and Methods).

The accuracy of our method was tested with measurements on freely diffusing taxol in thin (2 [mu]M) samples without microtubules. The data analysis yielded D = 1.3 + or - 0.1 x 10^sup -6^ cm^sup 2^/s, consistent with predicted values (Odde, 1998). The identical measurement in a flow cell (~100 [mu]M thick) was ~20% faster, consistent with the results of previous three-dimensional FRAP experiments (Berk et al., 1993).

Effect of bundle geometry on measured recovery times

Within a sample, bundles varied by an order of magnitude in apparent width, depth, and brightness. These features correlate with the number of microtubules and their packing density. Brighter, thicker bundles consistently recovered slower than dimmer, thinner bundles in the same sample (Fig. 4), indicating that taxol molecules diffuse perpendicular to the bundle axis. Another result that suggests lateral diffusion in the bundle is that the widths of the Gaussian intensity profiles in Fig. 3 did not substantially increase with time.

Effect of binding site density on measured recovery times

The density of unoccupied binding sites was varied by adjusting the taxol concentration in solution. Recovery times were averaged for a variety of bundles at each taxol concentration to overcome variations between bundles and meaningfully compare [tau] values for different binding site fill ratios.

For GMPCPP microtubules, the taxol concentrations used were from 25 pM to 2.5 [mu]M, which resulted in 0.2%-99% of the taxol binding sites filled (see Eq. 1). Recovery times for taxol on GMPCPP bundles ranged from ~1000 to 5000 s. As taxol concentration decreased, the average recovery time increased, indicating that mobility of taxol molecules is hindered by rebinding events on multiple unoccupied binding sites. The dependence is well-approximated by a power law, [tau]~[TAX]^sup -0.17+ or -0.03^. However, for taxol concentrations over 25 nM (>63% of binding sites occupied), [tau] was independent of taxol concentration (Fig. 5), implying that the open binding sites are no longer influencing taxol mobility.

of taxol on the density of unoccupied binding sites. Although taxol is able to move through the microtubule walls, the presence of binding sites along the microtubule is capable of hindering diffusion as long as unoccupied sites are less than ~7 nm apart. This work suggests that the microtubules could increase reaction rates by reducing the diffusive search inside the microtubule lumen.

We benefited from discussions with F. Brown, A. Ekani-Nkodo, J. Lew, and C. Santangelo. We are grateful to T. Mitchison for generous gifts of GMPCPP, and we also thank JJ. Correia for directing us to a commercial supplier of the same.

This work was supported partially by the National Science Foundation, through the CAREER program under grant 9985493, and through the Materials Research Laboratory (MRL) program under grant DMR00-80034; and partially by an Alfred P. Sloan Foundation Fellowship (to D.K.F.).

REFERENCES

Adam, G., and M. Delbruck. 1968. Reduction of dimensionality in biological diffusion processes. In Structural Chemistry in Molecular Biology. A. Rich and N. Davidson, editors. Freeman, San Francisco, pp. 198-215.

Alberts, B., D. Bray, J. Lewis, M. Raff, K. Roberts, and J. D. Watson. 1994. Molecular Biology of the Cell. Garland Publishing, New York.

Axelrod, D., D. E. Koppel, J. Schlessinger, E. Elson, and W. W. Webb. 1976. Mobility measurement by analysis of fluorescence photobleaching recovery kinetics. Biophys. J. 16:1055-1069.

Berg, O. G. 1978. On diffusion-controlled dissociation. Chem. Phys. 31:47-57.

Berg, O. G., R. B. Winter, and P. H. Vonhippel. 1981. Diffusion-driven mechanisms of protein translocation on nucleic acids. I. Models and theory. Biochemistry. 20:6929-6948.

Berk, D. A., F. Yuan, M. Leunig, and R. K. Jain. 1993. Fluorescence photobleaching with spatial Fourier analysis-measurement of diffusion in light-scattering media. Biophys. J. 65:2428-2436.

Derry, W. B., L. Wilson, and M. A. Jordan. 1995. Substoichiometric binding of taxol suppresses microtubule dynamics. Biochemistry. 34:2203-2211.

Dowd, D. R., and R. S. Lloyd. 1990. Biological significance of facilitated diffusion in protein-DNA interactions-applications to T4 endonuclease. V. Initiated DNA repair. J. Biol. Chem. 265:3424-3431.

Downing, K. H. 2000. Structural basis for the interaction of tubulin with proteins and drugs that affect microtubule dynamics. Annu. Rev. Cell Dev. Biol. 16:89-111.

Drewes, G., A. Ebneth, and E. M. Mandelkow. 1998. MAPs, MARKs and microtubule dynamics. Trends Biochem. Sci. 23:307-311.

Edelstein, A. L., and N. Agmon. 1997. Brownian simulation of many-particle binding to a reversible receptor array. J. Comput. Phys. 132:260-275.

Evangelio, J. A., M. Abal, I. Barasoain, A. A. Souto, M. P. Lillo, A. U. Acuna, F. Amat-Guerri, and J. M. Andreu. 1998. Fluorescent taxoids as probes of the microtubule cytoskeleton. Cell Motil. Cytoskeleton. 39:73-90.

Goode, B. L., M. Chau, P. E. Denis, and S. C. Feinstein. 2000. Structural and functional differences between 3-repeat and 4-repeat [tau]-isoformsimplications for normal [tau]-function and the onset of neurodegenerative disease. J. Biol. Chem. 275:38182-38189.

Heald, R., and E. Nogales. 2002. Microtubule dynamics. J. Cell Sci. 115:3-4.

Herendeen, D. R., G. A. Kassavetis, and E. P. Geiduschek. 1992. A transcriptional enhancer whose function imposes a requirement that proteins track along DNA. Science. 256:1298-1303.

Hyman, A. A., S. Salser, D. N. Drechsel, N. Unwin, and T. J. Mitchison. 1992. Role of GTP hydrolysis in microtubule dynamics-information from a slowly hydrolyzable analog, GMPCPP. Mol. Biol. Cell. 3:1155-1167.

Jack, W. E., B. J. Terry, and P. Modrich. 1982. Involvement of outside DNA sequences in the major kinetic path by which E. coli endonuclease locales and leaves its recognition sequence. Proc. Natl. Acad. Sci. USA. 79:4010-4014.

Kao, H. P., J. R. Abney, and A. S. Verkman. 1993. Determinants of the translational mobility of a small solute in cell cytoplasm. J. Cell Biol. 120:175-184.

Kapitza, H. G., G. McGregor, and K. A. Jacobson. 1985. Direct measurement of lateral transport in membranes by using time-resolved spatial photometry. Proc. Natl. Acad. Sci. USA. 82:4122-4126.

Lagerholm, B. C., and N. L. Thompson. 1998. Theory for ligand rebinding at cell membrane surfaces. Biophys. J. 74:1215-1228.

Li, Y. K., R. Edsall, P. G. Jagtap, D. G. I. Kingston, and S. Bane. 2000. Equilibrium studies of a fluorescent paclitaxel derivative binding to microtubules. Biochemistry. 39:616-623.

Lipowsky, R., S. Klumpp, and T. M. Nieuwenhuizen. 2001. Random walks of cytoskeletal motors in open and closed compartments. Physical Review Letters. 8710:art. no.-108101.

Mandelkow, E., and K. A. Johnson. 1998. The structural and mechano-chemical cycle of kinesin. Trends Biochem. Sci. 23:429-433.

Meurer-Grob, P., J. Kasparian, and R. H. Wade. 2001. Microtubule structure at improved resolution. Biochemistry. 40:8000-8008.

Nardone, G., J. George, and J. G. Chirikjian. 1986. Differences in the kinetic properties of Bamhi endonuclease and methylase with linear DNA substrates. J. Biol. Chem. 261:2128-2133.

Nieuwenhuizen, T. M., S. Klumpp, and R. Lipowsky. 2002. Walks of molecular motors in two and three dimensions. Europhys. Lett. 58:468-474.

Nogales, E., M. Whittaker, R. A. Milligan, and K. H. Downing. 1999. High-resolution model of the microtubule. Cell. 96:79-88.

Odde, D. 1998. Diffusion inside microtubules. Eur. Biophys. J. Biophys. Lett. 27:514-520.

Parekh, H., and H. Simpkins. 1997. The transport and binding of taxol. Gen. Pharmacol. 29:167-172.

Ramakrishnan, A., and A. Sadana. 1999. Analysis of analyte-receptor binding kinetics for biosensor applications: an overview of the influence of the fractal dimension on the surface on the binding rate coefficient. Biotechnol. Appl. Biochem. 29:45-57.

Renkin, E. M. 1954. Filtration, diffusion, and molecular sieving through porous cellulose membranes. J. Gen. Physiol. 38:225-243.

Ricchetti, M., W. Metzger, and H. Heumann. 1988. One-dimensional diffusion of Escherichia coli DNA-dependent RNA polymerase-a mechanism to facilitate promoter location. Proc. Natl. Acad. Sci. USA. 85:4610-4614.

Riggs, A. D., S. Bourgeoi, and M. Cohn. 1970. Lac repressor-operator interaction. III. Kinetic studies. J. Mol. Biol. 53:401-417.

Rivas, G., J. A. Fernandez, and A. P. Minton. 1999. Direct observation of the self-association of dilute proteins in the presence of inert macromolecules at high concentration via tracer sedimentation equilibrium: theory, experiment, and biological significance. Biochemistry. 38:9379-9388.

Robinson, J., and Y. Engelborghs. 1982. Tubulin polymerization in dimethylsulfoxide. J. Biol. Chem. 257:5367-5371.

Sosa, H., D. P. Dias, A. Hoenger, M. Whittaker, E. Wilson-Kubalek, E. Sablin, R. J. Fletterick, R. D. Vale, and R. A. Milligan. 1997. A model for the microtubule-NCD motor protein complex obtained by cryo-electron microscopy and image analysis. Cell. 90:217-224.

Sung, J., K. J. Shin, and S. Lee. 1997. Many-particle effects on the relaxation kinetics of fast reversible reactions of the type A+B reversible arrow C. J. Chem. Phys. 107:9418-9436.

Surby, M. A., and N. O. Reich. 1996. Facilitated diffusion of the EcoRI DNA methyltransferase is described by a novel mechanism. Biochemistry. 35:2209-2217.

Thompson, N. L., and D. Axelrod. 1983. Immunoglobulin surface-binding kinetics studied by total internal reflection with fluorescence correlation spectroscopy. Biophys. J. 43:103-114.

Tran, P. T., P. Joshi, and E. D. Salmon. 1997. How tubulin subunits are lost from the shortening ends of microtubules. J. Struct. Biol. 118: 107-118.

Tsay, T, T., and K. A. Jacobson. 1991. Spatial Fourier analysis of video photobleaching measurements-principles and optimization. Biophys. J. 60:360-368.

Vigers, G. P. A., M. Coue, and J. R. Mclntosh. 1988. Fluorescent microtubules break up under illumination. J. Cell BM. 107:1011-1024.

Vilfan, A., E. Frey, and F. Schwabl. 2001. Relaxation kinetics of biological dinier adsorption models. Europhys. Lett. 56:420-426.

Vonhippel, P. H., and O. G. Berg. 1989. Facilitated target location in biological systems. J. Biol. Chem. 264:675-678.

Winter, R. B., and P. H. Vonhippel. 1981. Diffusion-driven mechanisms of protein translocation on nucleic acids. II. The Escherichia coli repressor-operator interaction-equilibrium measurements. Biochemistry. 20:6948-6960.

Jennifer L. Ross and D. Kuchnir Fygenson

Physics Department, University of California, Santa Barbara, California 93106

Submitted August 19, 2002, and accepted for publication January 29, 2003.

Address reprint requests to Deborah Kuchnir Fygenson, University of California at Santa Barbara, Santa Barbara, CA 93106-9530. Tel: 805-893-2449; Fax: 805-893-3307; E-mail: deborah@physics.ucsb.edu.

(C) 2003 by the Biophysical Society

0006-3495/03/06/3959/09 $2.00

Copyright Biophysical Society Jun 2003
Provided by ProQuest Information and Learning Company. All rights Reserved